Introduction to Fractal Analysis
How would you characterize the images on this page? Describing them using traditional features such as "size" and overall "shape" wouldn't really say much, although it could be very informative for some other forms, such as when characterizing "a 10 cm, round orange" or "a 300 cm x 100 cm oblong watermelon", for instance. But using such descriptors for the images here would oversimplify the detail in their patterns. Describing these patterns using the terms of fractal analysis with FracLac, however, can convey some of the complexity inherent in their design.
 These images show diffusion limited aggregation, which is a type of fractal growth that can be analyzed with FracLac. The images were generated with the DLA Generator for ImageJ.
These images show diffusion limited aggregation, which is a type of fractal growth that can be analyzed with FracLac. The images were generated with the DLA Generator for ImageJ.
What is Fractal Analysis?
Fractal analysis is a contemporary method of applying nontraditional mathematics to patterns that defy understanding with traditional Euclidean concepts.
In essence, it measures complexity using the fractal dimension.
The field was developed to describe computer-generated fractals such as the diffusion limited aggregates shown on this page, but fractals are not necessarily computer-generated images. Rather, whereas we see Euclidean geometry in familiar shapes like oranges and watermelons, we see fractal geometry in familiar forms like meandering coastlines, growing crystals, and swirling galaxies.
Fractal Patterns
Fractals are not necessarily physical forms - they can be spatial or temporal patterns, as well. In general, fractals can be any type of infinitely scaled and repeated pattern. In this regard, it is important to be aware that theoretical fractals are abstractions, but the subjects of fractal analysis, such as digital images limited by screen resolution, are generally not true fractals in the strictest sense. Similarly, the so-called fractals typically found in nature are not infinitely scaled, thus, like finite computer generated patterns, are generally only approximations to fractals in the strictest sense.
Imagine a real fractal.
The computer generated pattern shown here was constructed to emulate a 32-segment quadric fractal.
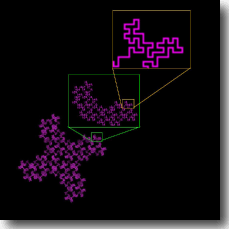 It's essential pattern is scaled and repeated to make the whole figure, but as illustrated, the scaling is not infinite - as highlighted in the upper box, it ends when the pattern becomes 1 pixel wide, a limit imposed by pixels on a computer screen.
It's essential pattern is scaled and repeated to make the whole figure, but as illustrated, the scaling is not infinite - as highlighted in the upper box, it ends when the pattern becomes 1 pixel wide, a limit imposed by pixels on a computer screen.
Click on the image to see it in higher resolution or click here to read about digital images of fractals.
Fractal Analysis in Practice
Notwithstanding the fact that what we actually investigate by fractal analysis merely approximates fractals, this sort of analysis is very useful. Investigators use different types of fractal analysis to study a host of otherwise intractable phenomena including the complex geometries of many types of biological cells (Kam et al. 2009) and complex patterns such as tree growth, river paths, tumour growth (Cross 1997), heart rates (Huikiri & Stein 2012), diabetic retinopathy (Karperien et al. 2008), gene expression (Aldrich et al 2010), forest fire progression (Turcotte et al. 2002), economic trends, and cellular differentiation in space and time (Waliszewski & Konarski 2002) (search PubMed or see FracLac Citations for more information). The next page discusses fractal analysis in more detail.